Nature offers many means to produce power. Most are through combustion, mechanical movement, photosynthesis or electrochemical reaction as in a battery. An electrochemical reaction produces a voltage potential, and multiplying the voltage by the current that flows when closing the electrical circuit provides power. Power is measured in watts in honor of James Watt, the eighteenth-century developer of the steam engine.
The most simplistic manifestation of a battery is a lemon. Driving a zinc-plated nail and a copper coin into a lemon creates a voltage. This quasi battery does not deliver much power; its current delivery system is very poor and any electrical load causes the voltage to collapse.
All power sources have limitations and the energy drawn must be harnessed carefully so as not to exceed the permitted loading. An analogy is a bicycle rider who chooses the best gear ratio to transfer energy into propulsion. On a flat road a high gear provides fast movement with moderate pedal action, and this can be compared to high voltage. Climbing a hill with the same pedaling action increases the torque, and in our analogy this corresponds to high current. The pedal force the rider exerts relates to power in watt (W); the endurance before exhaustion defines energy in watt/hours (Wh).

Energy is the product of power and time, measured in watt-hours (Wh); power is the flow of energy at any one time, measured in watts.
A battery is rated in ampere/hours (Ah); it specifies how much current a pack can deliver in an hour. Like fluid in a container, the energy can be dispensed slowly over a long period of time or rapidly in a short time. The amount of liquid a container holds is analogous to the energy in a battery; how quickly the liquid is dispensed is analogous to power.
An alkaline battery has low power with a relatively high specific energy (capacity). See BU-106: Primary Batteries This lends itself well for a flashlight or a similar light load. In comparison, most rechargeable batteries have high load capabilities to drive power tools and crank internal combustion engines but these batteries have lower capacities than the primary counterpart.
The relationship between energy and power can best be represented in a Ragone plot. Named after David V. Ragone, the Ragone plot places the energy in Wh on the horizontal x axis and power in W on the vertical y axis. The derived power curve provides a clear demarcation line of what level of power a battery can deliver. The Ragone plot is logarithmic, which enables displaying performance profiles of extremely high and low power. Some table may reverse the W and Wh positioning.
Figure 2 illustrates the Ragone plot reflecting the discharge energy and discharge power of four lithium-ion systems packaged in 18650 cells. The diagonal lines across the field disclose the length of time the battery cells can deliver energy at various loading conditions. The battery chemistries featured are the most common power-based lithium-ion systems, which include lithium-iron phosphate (LFP), lithium-manganese oxide (LMO), and nickel manganese cobalt (NMC)
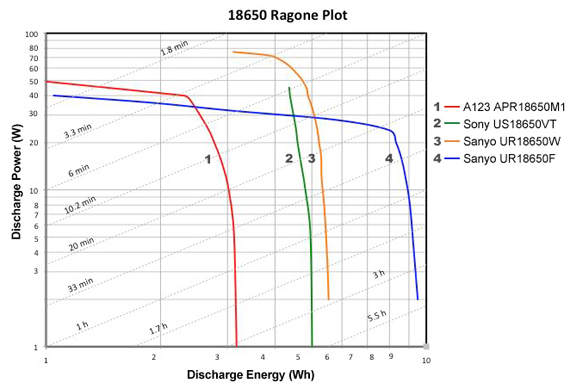
Four Li-ion systems are compared for discharge power and energy as a function of time.
Legend: The A123 APR18650M1 is a lithium iron phosphate (LiFePO4) with 1,100mAh and a continuous discharge current of 30A. The Sony US18650VT and Sanyo UR18650W are manganese–based Li-ion cells of 1500mAh each with a continuous discharge current of 20A. The Sanyo UR18650F is a 2,600mAh cell for a moderate 5A.discharge. This cell provides the highest discharge energy but has the lowest discharge power.
The physical dimensions of a battery are specified by volume in liter (l) and weight in kilogram (kg). Adding dimension and weight enables rating a battery in specific energy in Wh/kg, power density in Wh/l and specific power in W/kg. Most batteries are rated in Wh/kg, revealing how much energy a given weight can generate. Wh/l denotes watt/hours per liter.
The Sanyo UR18650F has the highest specific energy and can power a laptop or e-bike for many hours at a moderate load. The Sanyo UR18650W, in comparison, has a lower specific energy but can supply a current of 20A. The A123 has the lowest specific energy but offers the highest power capability by delivering 30A of continuous current.
The Ragone plot helps choosing the best Li-ion system to satisfy maximum discharge power and optimal discharge energy as a function of discharge time. If an application calls for very high discharge current, the 3.3 minute diagonal line on the chart points to the A123 (Battery 1) as a good pick; it can deliver up to 40 Watts of power for 3.3 minutes. The Sanyo F (Battery 4) is slightly lower and delivers about 36 Watts. Focusing on discharge time and following the 33 minute discharge line further down, Battery 1 (A123) only delivers 5.8 Watts for 33 minutes before the energy is depleted whereas the higher capacity Battery 4 (Sanyo F) can provide roughly 17 Watts for the same time; its limitation is lower power.
Battery manufacturers take the Ragone snapshot on new cells, a condition that is only valid for a short time. When calculating power and energy thresholds, design engineers must consider battery fade caused by cycling and aging. Design battery operated systems that still provide full function with a battery that has faded to 70 or 80 percent. A further consideration is temperature as batteries lose power when cold. The Ragone plot does not show these discrepancies and the design engineer must take these less-than-ideal-conditions into consideration by studying the manufacturer’s specifications.
It should be noted that loading a battery to its full power handling increases stress and shortens the life. When a high current draw is needed continuously, the battery pack should be made larger. Tesla does this with their Model S cars by doubling and tripling the battery size compared to other EVs; BMW i3 uses a smaller but more rugged Li-ion system. An analogy can be drawn with a heavy truck that is fitted with a large diesel engine to provide long and durable service as opposed to installing a souped-up sports car engine with similar horsepower.
The Ragone plot is also suitable to calculate power requirements of other energy sources and storage devices, such as capacitors, flywheels, flow batteries and fuel cells. Fuel cells and internal combustion engines drawing fuel from a tank causes a conflict in that energy-delivery can be made continuous. This distorts the Wh measurements of a self-contained battery (or the bicycle rider) to determine the available intrinsic energy before recharging is required.
Similar plots are also deployed to establish the optimal energy/power ratio and loading condition of renewable power sources such as solar cells and wind turbines. An example of such a chart is the maximum power point tracking (MPPT) used on charge controllers to charge batteries from renewable resources. See BU-413: Charging with Solar, Turbine MPPT allows optimal power transfer without overloading the source when the supply is low during fringe conditions Also see Figure 2 in specific energy and specific power of rechargeable batteries table. See BU-103: Global Battery Markets
References
[1] Courtesy of Exponent


I want battery life to be okey